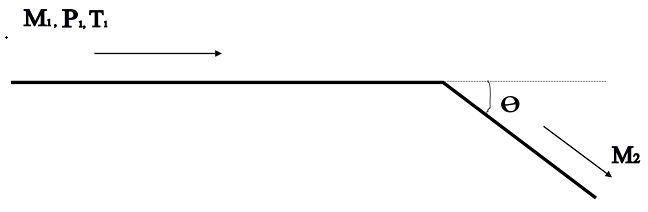
Consider the inviscid,adiabatic flow of air at free stream conditions M_{1}=2,P_{1}=1\;atm and T_{1}=288\;K around a sharp expansion corner \left ( \theta =20^{\circ} \right ). Assume air to be calorically perfect with \gamma =1.4. What is the Mach number M_{2}, downstream of the expansion corner ?
Consider the inviscid,adiabatic flow of air at free stream conditions M_{1}=2,P_{1}=1\;atm and T_{1}=288\;K around a sharp expansion corner \left ( \theta =20^{\circ} \right ) as shown below. The Prandtl-Meyer function \nu, is given as a function of Mach number M , as
\nu (M)=\sqrt{\frac{\gamma +1}{\gamma -1}}tan^{-1}\sqrt{\frac{\gamma -1}{\gamma +1}\left ( M^{2} -1\right )}-tan^{-1}\sqrt{M^{2}-1}
Assume air to be calorically perfect with \gamma =1.4 . What is the Mach number M_{2}, downstream of the expansion corner ?
Prandtl-Meyer expansion theory tells that \theta =\nu \left ( M_{2} \right )-\nu \left ( M_{1} \right )
Here
\nu \left ( M_{1} \right )=\sqrt{\frac{2.4}{0.4}}tan^{-1}\sqrt{\frac{0.4}{2.4}\times 3}-tan^{-1}\sqrt{3} \\=26.38^{\circ}
\nu \left ( M_{2} \right )=\theta +\nu \left ( M_{1} \right ) \\=20^{\circ}+26.38^{\circ} \\=46.35^{\circ}
On putting the value of \nu (M_{2}) in the Prandtl-Meyer function we get
46.35^{\circ}=\sqrt{\frac{2.4}{0.4}}tan^{-1}\sqrt{\frac{0.4}{2.4}\left ( M_{2}^{2}-1 \right )}-tan^{-1}\sqrt{M_{2}^{2}+1}
\Rightarrow M_{2}=2.83 approximately.