624
Points
Questions
72
Answers
119
-
Asked on 8th October 2021 in Aerodynamics.
Pressure is defined as force per unit area. In fluid pressure on a surface is the normal force acting per unit area due to time rate of change of momentum of the gas molecules which is impacting the surface.
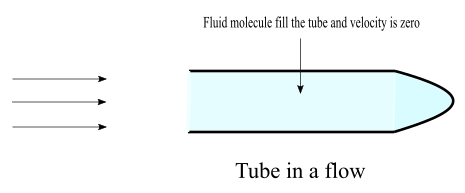
The pressure due to the pure random motion of the molecules of the gas is the static pressure. Whenever a body is in a flow, the fluid will get an obstruction at the surface of the body and the velocity of the gas molecule will be zero. If a body is a tube with one end closed, the fluid molecules will fill the tube and its velocity will be zero, that the fluid will stagnate.
At the opening of the tube the fluid molecules sees obstruction. Therefore upcoming fluid molecules slows down and reduces to zero velocity. Any point in a fluid flow where flow velocity is zero is called the stagnation point. As velocity is decreased so pressure is increased. Therefore, pressure at stagnation point is larger than the static pressure.
The pressure at the stagnation point is called the stagnation pressure or total pressure.
Therefore, there are two types of pressure in a fluid flow, static pressure and total or stagnation pressure. Static pressure is the pressure which is felt when a fluid has a velocity and stagnation pressure is the pressure that is felt when flow velocity is reduced to zero.
If a flow is isentropic in between two points then the total pressure is constant at that points. An isentropic process is a process in which entropy remains constant. An isentropic process is both reversible and adiabatic.
The temperature of a gas is directly proportional to the average kinetic energy of the gas molecules. Therefore, a gas which has a high temperature, the molecules are moving randomly at a very high speed. A gas in low-temperature, there is a slow random motion of the molecules as compared to the high temperature gas.
The temperature of a fluid element when it is bought to rest adiabatically is called the total temperature. Stagnation temperature is the temperature when a moving flow is isentropically brought to rest. At the stagnation point total temperature and stagnation temperature have the same value.
- 1361 views
- 1 answers
- 0 votes
-
Asked on 27th August 2021 in Aeronautics.
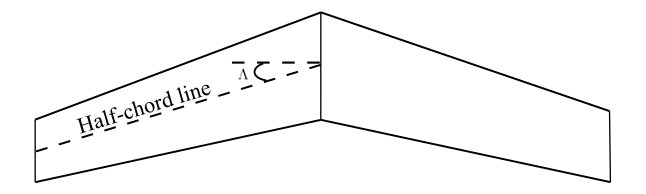
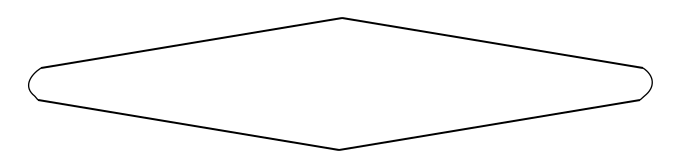 High aspect ratio straight wing
High aspect ratio straight wingUsing Helmbold’s equation, for calculating the lift slope for straight wing, a = \frac{a_{0}}{\sqrt{1+\left ( \frac{a_{0}}{\pi AR} \right )^{2}}+\left ( \frac{a_{0}}{\pi AR} \right )} Lift slope for the airfoil = a_{0} = 0.15\,/degree = 8.5944\,/radian.
Therefore,
a = \frac{8.5944}{\sqrt{1+\left ( \frac{8.5944}{\pi \left ( 6 \right )} \right )^{2}}+\left ( \frac{8.5944}{\pi \left ( 6 \right )} \right )}=1.4569\,per\,radian
Using Helmbold’s equation, for calculating the lift slope for a swept wing ,a = \frac{a_{0}cos\Lambda }{\sqrt{1+\left ( \frac{a_{0}cos\Lambda}{\pi AR} \right )^{2}}+\left ( \frac{a_{0}cos\Lambda}{\pi AR} \right )}Here, \Lambda = 30^{0}, is the sweep angle of the wing referenced to the half-chord line. Therefore, a = \frac{8.5944cos30^{0}}{\sqrt{1+\left ( \frac{8.5944cos30^{0}}{\pi \left ( 6 \right )} \right )^{2}}+\left ( \frac{8.5944cos30^{0}}{\pi \left ( 6 \right )} \right )}=1.4436\,per\,radianOn comparing the lift slopes of straight wing and swept wing, we can see that there is a reduction in lift slope for the swept wing.
Wing sweep is decreasing the lift slope, and it affects lift slope to a larger degree for a high aspect ratio wings than for a lower aspect ratio wings.
- 1429 views
- 1 answers
- 0 votes
-
Asked on 26th July 2021 in Aeronautics.
Span is the wingspan of the airplane. It is the distance from one wing tip to other. It is measured in a straight line and is independent of the wing shape and sweep. Lift per unit span produced by a wing is given as {L}’ = \frac{1}{2} \rho _{\infty}V_{\infty}^{2} c\left ( 1 \right )c_{l}. Here, \frac{1}{2} \rho_{\infty}V_{\infty}^{2} is called the dynamic pressure , q_{\infty} . Coffeicent of lift, c_{l}, for this airfoil will be c_{l} = \frac{{L}’}{q_{\infty}c\left ( 1 \right )} \Rightarrow c_{l} = \frac{1400}{\left (\frac{1}{2} \times 1.225 \times 70^{2} \right )\left ( 2.3 \right )\left ( 1 \right )}\Rightarrow c_{l} = 0.203Therefore, coefficient of lift for this airfoil is 0.203. For, NACA 0012 airfoil from the lift curve -slope for this airfoil, the angle of attack is 2^{\circ} .
- 1307 views
- 1 answers
- 0 votes
-
Asked on 6th July 2021 in Aerodynamics.
Pressure coefficient is given as C_{p}=\frac{p_{1} – p_{\infty }}{q_{\infty }}This is used for incompressible to compressible flow. However, for incompressible flow, this expression can be used in terms of velocity alone, on applying the Bernoulli’s equation. On using Bernoulli’s equation for two points in a flow, p_{1}+\frac{1}{2}\rho V_{1}^{2} = p_{\infty } + \frac{1}{2}\rho v_{\infty }^{2}p_{1}-p_{\infty} = \frac{1}{2} \rho \left ( V_{\infty}^{2}-V_{1}^{2} \right )Considering p_{1} as the pressure at a point on the wing of the aircraft, and p_{\infty} as freestream pressure, coefficient of pressure is,C_{p} = \frac{p_{1} – p_{\infty }}{q_{\infty}}=\frac{\frac{1}{2}\rho \left ( V_{\infty}^{2}-V_{1}^{2} \right )}{\frac{1}{2}\rho V_{\infty}^{2}}=1-\left ( \frac{V_{1}}{V_{\infty}} \right )^{2}\Rightarrow C_{p} = 1 – \left ( \frac{140}{100} \right )^{2}=-0.96
- 1140 views
- 1 answers
- 0 votes
-
RE: Find the velocity vector and its magnitude at a point (pleft ( 3,2,4 right )) at (t=5 ) seconds.Asked on 20th May 2021 in Fluid mechanics.
Velocity components are u=\left ( 6x^{2}y+t \right ); v=\left ( 3yx+t^{2}+3 \right ); w=\left ( 3+3t^{2}y \right)
Velocity vector is given as,
\vec{V}=u\hat{i}+v\hat{j}+w\hat{k}At point (3,2,4) and at t=5, velocity components will be,
u=\left ( 6x^{2}y+t \right )=\left ( 6\left ( 3 \right )^{2}2+5 \right )=113v=\left ( 3yx+t^{2}+3 \right )=\left ( \left ( 3\times 2\times 3 \right )+25+3 \right )=\left ( 18+25+3 \right )=46w=\left ( 3+3t^{2}y \right )=\left ( 3+\left ( 3\left ( 5 \right )^{2}2 \right ) \right )=\left ( 3+150 \right )=153
Therefore, velocity vector is,
\vec{V}=113\hat{i}+46\hat{j}+153\hat{k}Velocity magnitude is, V=\sqrt{u^{2}+v^{2}+w^{2}}=\sqrt{113^{2}+46^{2}+153^{2}}=195.69\,m/s- 1249 views
- 1 answers
- 0 votes
-
Asked on 7th May 2021 in Fluid mechanics.
The stream function for the flow field is \psi =2x^{2}-y^{2}Velocity component u and v of the two-dimensional flow field is u=\frac{\partial \psi }{\partial y},v=-\frac{\partial \psi }{\partial x}Therefore,u=\frac{\partial \psi }{\partial y}=\frac{\partial }{\partial y}\left ( 2x^{2}-y^{2} \right ) =\frac{\partial }{\partial y}\left ( 2x^{2} \right )-\frac{\partial }{\partial y}\left ( y^{2} \right )=0-2y=-2yv=-\frac{\partial \psi }{\partial x} =-\frac{\partial }{\partial x}\left ( 2x^{2}-y^{2} \right ) =-\frac{\partial }{\partial x}\left ( 2x^{2} \right )+\frac{\partial }{\partial x}\left ( y^{2} \right )=-4x+0=-4x Therefore, at point (2,3),u=-2y=-2\left ( 3 \right )=-6\\v=-4x=-4\left ( 2 \right )=-8Velocity, V=-6i-8j
Magnitude of velocity = \sqrt{u^{2}+v^{2}}=\sqrt{\left ( -6 \right )^{2}+\left ( -8\right)^{2}}=\sqrt{36+64}=10- 1242 views
- 1 answers
- 0 votes
-
Asked on 19th April 2021 in Aerodynamics.
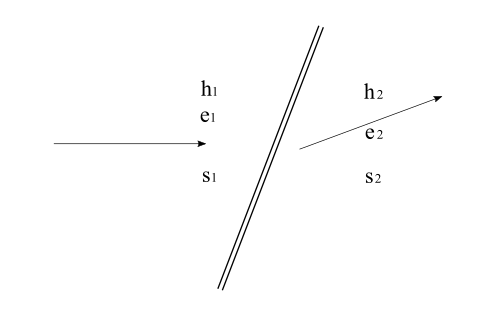 Flow passing through a shock wave
Flow passing through a shock waveSpecific heat at constant pressure,
c_{p}=\frac{\gamma R}{\gamma -1}=\frac{\left ( 1.4 \right )\left ( 287 \right )}{\left ( 1.4-1 \right )}=1004.5\,J/\left (kg.K \right )
Specific heat at condtant volume,
c_{v}=\frac{R}{\gamma -1}=\frac{287}{0.4}=717.5\,J/kg.K
Enthalpy change across the shock wave,
h_{2}-h_{1}=c_{p}\left ( T_{2}-T_{1} \right )=1004.5\left ( 700-300 \right )=4.018\times 10^{5}\,J/kg
Internal energy change across the shock wave,
e_{2}-e_{1}=c_{v}\left ( T_{2}-T_{1} \right )=717.5\left ( 700-300 \right )=2.87\times 10^{5}\,J/kg
Entopy change across the shock wave,
s_{2}-s_{1}=c_{p}ln\left ( \frac{T_{2}}{T_{1}} \right ) -Rln\left ( \frac{p_{2}}{p_{1}} \right )=1004.5ln\left ( \frac{700}{300} \right )-287ln\left ( \frac{9}{1} \right )=220.51\,J/kg.K- 1338 views
- 1 answers
- 0 votes
-
Asked on 5th April 2021 in Aerodynamics.
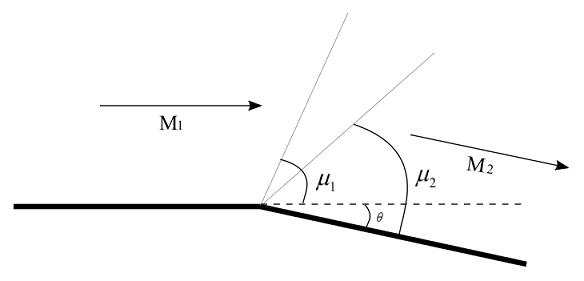
Downstream Mach number can be calculated from Prandtl-Meyer function
\nu (M)=\sqrt{\frac{\gamma +1}{\gamma -1}}tan^{-1}\sqrt{\frac{\gamma -1}{\gamma +1}\left ( M^{2}-1 \right )}-tan^{-1}\sqrt{M^{2}-1}For a Mach number of 2.2, \nu \left ( M_{1} \right )=31.73^{^{\circ}}
\theta =\nu \left ( M_{2} \right )-\nu \left ( M_{1} \right )
\Rightarrow \nu \left ( M_{2} \right )=\theta + \nu \left ( M_{1} \right ) = 25^{\circ}+31.73^{\circ}=56.73^{\circ}
for, \nu \left ( M_{2} \right )=56.73^{^{\circ}}, Mach number M_{2}=3.389
From Isentropic flow properties for, M_{1}=2.2
\frac{p_{01}}{p_{1}}=10.69 and
\frac{T_{01}}{T_{1}}=1.968For, M_{2}=3.389
\frac{p_{02}}{p_{2}}=65.0798 and
\frac{T_{02}}{T_{2}}=3.297Total pressure and total temperature is constant through the expansion wave, so
p_{01}=p_{02} and T_{01}=T_{02}
Therefore,
p_{2}=\left ( \frac{p_{2}}{p_{02}} \right )\left ( \frac{p_{01}}{p_{1}} \right )p_{1}=\left ( \frac{1}{65.0798} \right )\left ( 10.69 \right )0.8=0.1314\,atmT_{2}=\left ( \frac{T_{2}}{T_{02}} \right )\left ( \frac{T_{01}}{T_{1}} \right )T_{1}=\left ( \frac{1}{3.297} \right )\left ( 1.968 \right )360=214.886\,K
p_{2}=\rho _{2}RT_{2}
\Rightarrow 0.1314=\rho _{2}\left ( 287 \right )\left ( 214.886\,K \right )
\Rightarrow \rho _{2}=\frac{0.1314}{\left ( 287 \right )\left ( 214.886 \right )}=2.13\times 10^{-6}\,kg/m^{3}
Since,
p_{01}=p_{02}\Rightarrow p_{02}=\left ( \frac{p_{01}}{p_{1}} \right )\left ( p_{1} \right )=\left ( 10.69 \right )\left ( 0.8 \right )=8.552\,atmAlso,
T_{01}=T_{02}\Rightarrow T_{02}=\left ( \frac{T_{01}}{T_{1}} \right )\left ( T_{1} \right )=\left ( 1.968 \right )\left ( 360 \right )=708.48\,K
Mach angle is given by \mu = Sin^{-1}\left ( \frac{1}{M} \right )
Therefore Mach angle \mu _{1} made by upstream Mach wave for M_{1}=2.2 is 27.04^{\circ}
Mach angle \mu _{2} made by downstream Mach wave forM_{2}=3.389 is 17.16^{\circ}
and, angle made by forward Machline is 27.04^{\circ} and angle made by the rearward Machline is 17.16^{\circ}-25^{\circ}=-7.84^{\circ}
- 1103 views
- 1 answers
- 0 votes
-
Asked on 19th March 2021 in Aerodynamics.
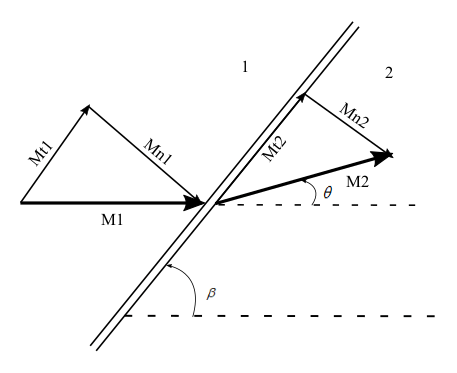
Normal component of the upstream Mach number to the oblique shock wave,
M_{n1}=M_{1}Sin\beta =4\left ( Sin30^{\circ} \right )=2
From Normal shock wave properties table, for M_{n1}=2
\frac{p_{2}}{p_{1}}=4.5, \frac{T_{2}}{T_{1}}=1.687, \frac{p_{02}}{p_{01}}=0.7209, M_{n2}=0.5774
At an altitude of 9000\, m , p_{1}=3.08\times 10^{4} N/m^{2} , T_{1}=229.74K
Therefore,
P_{2}=\left ( \frac{P_{2}}{P_{1}} \right )P_{1}=\left ( 4.5 \right )\left ( 3.08\times 10^{4} \right)= 13.86\times 10^{4} N/m^{2}
T_{2}=\left ( \frac{T_{2}}{T_{1}} \right )T_{1}=\left ( 1.687 \right )229.74=387.571K
From the \theta -\beta -M diagram , for, wave angle of \beta =30^{\circ} and Mach number of 4, deflection angle, \theta =17.7^{\circ}.
Therefore,
M_{2}=\frac{M_{n2}}{Sin\left ( \beta -\theta \right )}=\frac{0.5774}{Sin\left ( 30^{\circ} -17.7^{\circ}\right )}=2.710
From the table of isentropic flow properties , for
M_{1}=4,\frac{p_{01}}{p_{1}}=151.8,\frac{T_{01}}{T_{1}}=4.2
Total pressure,
p_{02}=\left ( \frac{p_{02}}{p_{01}} \right )\left ( \frac{p_{01}}{p_{1}} \right )p_{1}=\left ( 0.0709 \right )\left ( 151.8 \right )\left ( 3.08\times 10^{4} \right )=331488.696N/m^{2}
Total temperature,
T_{02}=T_{01}=\left ( \frac{T_{01}}{T_{1}} \right )T_{1}=4.2\times 229.74K=964.908K
- 1350 views
- 1 answers
- 0 votes
-
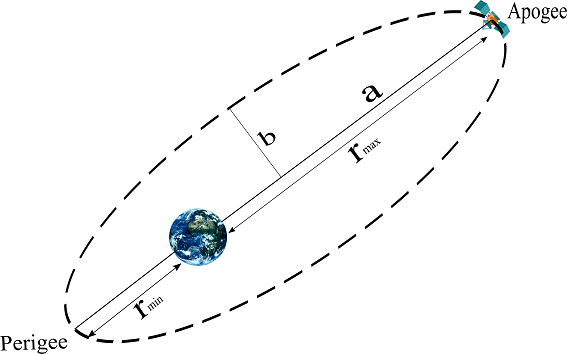
Asked on 9th March 2021 in Space dynamics.
\frac{{{r_{\max }}}}{{{r_{\min }}}} = \frac{{1 + e}}{{1 – e}} = \frac{{1 + 0.0014}}{{1 – 0.0014}} = 1.0028
\Rightarrow {r_{\max }} = {r_{\min }}\left( {1.0028} \right)
{r_{\min }} = {\rm{radius}}\,{\rm{of}}\,{\rm{earth}}\,{\rm{ + }}\,{\rm{altitude}}\,{\rm{above}}\,{\rm{earth’s}}\,{\rm{surface}}
\Rightarrow {{\rm{r}}_{\min }} = 6.4 \times {10^6} + 0.4 \times {10^6} = 6.8 \times {10^6}\,m
\Rightarrow {r_{\max }} = 6.8 \times {10^6}\left( {1.0028} \right) = 681904\,m = 6.81904 \times {10^6}\,m
Therefore satellite’s altitude at apogee is
6.81904 \times {10^6} – 6.4 \times {10^6} = 419040\,m = 419.04\,km
Time period of the satellite is given as
{T^2} = \frac{{4{\pi ^2}}}{{{k^2}}}{a^3}
{k^2} = G{M_{earth}} = 3.986 \times {10^{14}}\,{m^3}/{s^2}
\Rightarrow a = {\rm{semi}}\,{\rm{major}}\,{\rm{axis}}\,{\rm{ = }}\frac{{{r_{\max }} + {r_{\min }}}}{2} = \frac{{6.81904 \times {{10}^6} + 6.8 \times {{10}^6}}}{2} = 6.80952 \times {10^6}\,m
{T^2} = \frac{{4{\pi ^2}}}{{3.986 \times {{10}^{14}}}} \times {\left( {6.80952 \times {{10}^6}} \right)^3}
\Rightarrow T = 5592.242\,s = 1.5534\,hr
{V_{perigee}} = {r_{\min }}\dot \theta
\dot \theta = \frac{h}{{{{\left( {{r_{\min }}} \right)}^2}}}
{h^2} = {r_{\min }}\left( {1 + e} \right){k^2}
\Rightarrow {h^2} = \left( {6.8 \times {{10}^6}} \right)\left( {1 + 0.0014} \right)3.986 \times {10^{14}}
\Rightarrow h = 5.20987 \times {10^{10}}
Therefore,
\dot \theta = \frac{{5.20987 \times {{10}^{10}}}}{{{{\left( {6.8 \times {{10}^6}} \right)}^2}}} = 0.0011267\,rad/s
{V_{perigee}} = \left( {6.8 \times {{10}^6} \times 0.0011267} \right) = 7661.56\,m/s = 7.66156\,km/s
- 1219 views
- 1 answers
- 0 votes