Find the velocity of an airplane flying at a standard sea level, if the static pressure at the throat of venturi is 100 000 N/m^{2}.
Find the velocity of an airplane flying at a standard sea level, if the static pressure at the throat of venturi is 100 000 N/m^{2}.
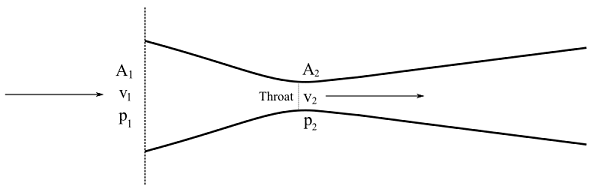
A venturi is a device which is used to measure the airspeed. It is a converging diverging duct. The inlet pressure and velocity of the duct is p_{1} and v_{1}. At the throat of the duct velocity increases to the maximum, v_{2} and pressure decrease to minimum, p_{2}. In the divergent section of the duct, velocity decreases and pressure increases. The inlet and throat area of the duct are A_{1} and A_{2}.
Velocity of air is calculated using Bernoulli’s equation, which isv_{1}=\sqrt{\frac{2\left ( p_{1} – p_{2} \right )}{\rho \left [ \left ( \frac{A_{1}}{A_{2}} \right )^{2}-1\right ]}} Here, p_{1} is the atmospheric pressure, which is 101325\,N/m^{2}, p_{2}=100000\,N/m^{2},\frac{A_{2}}{A_{1}} = 0.8.On putting the values, v_{1}=\sqrt{\frac{2\left ( 101325-100000 \right )}{1.225\left [ \left ( \frac{1}{0.8} \right )^{2}-1\right ]}}\Rightarrow v_{1}=62.015\,m/sTherefore, velocity of the airplane is 62.015\,m/s.